Question

Refer to speeds at which cars pass through a checkpoint on the highway. Assume the speeds are normally distributed with a population mean of 61 miles per hour and a population standard deviation of 4 miles per hour. Calculate the probability that the next car passing will be travelling more than 66 miles per hour.

Answer 1

Probability that the next car passing will be travelling more than 66 miles per hour is 0.10565.

Explanation:

We are given that the the speeds are normally distributed with a population mean of 61 miles per hour and a population standard deviation of 4 miles per hour.

Let X = speed of car

The z-score probability distribution for normal distribution is given by;

Z =
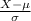`( X-\mu)/(\sigma)} }` ~ N(0,1)

where,
`\mu` = population mean speed = 61 miles per hour

`\sigma` = standard deviation = 4 miles per hour

The Z-score measures how many standard deviations the measure is away from the mean. After finding the Z-score, we look at the z-score table and find the p-value (area) associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X.

Now, Probability that the next car passing will be travelling more than 66 miles per hour is given by = P(X > 66 miles per hour)

P(X > 66) = P(
`( X-\mu)/(\sigma)} }` >
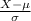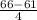`( 66-61)/(4)} }` ) = P(Z > 1.25) = 1 - P(Z
`\leq` 1.25)

= 1 - 0.89435 = 0.10565

So, in the z table the P(Z
`\leq` x) or P(Z < x) is given. So, the above probability is calculated by looking at the value of x = 1.25 in the z table which has an area of 0.89435.

Hence, the probability that the next car passing will be travelling more than 66 miles per hour is 0.10565.