Question

Solve tan(x)(tanx+1)=0

Answer 1

Answer:
`x=n\pi , (3\pi )/(4) +n\pi`

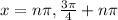
Explanation:

Since we have a product here, either term can be zero. We can individually set each term equal to zero:

`tan(x)=0`

`tan(x)+1=0`

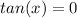
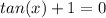
Beginning with the first term. It is saying "tangent of what gives zero". Well, tangent of zero gives zero. The value pi also gives zero:

`tan(0)=0`

`tan(\pi )=0`

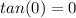
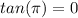
We can generalize this by saying 'x' is equal to pi multiplied by an integer 'n', where 'n' is 0, 1, 2, 3...

`x=n\pi`

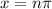
Moving on to the second term, lets subtract that 1 to the other side to get:

`tan(x)=-1`

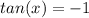
Tangent of what gives -1? That would be 3pi/4. Tangent is the ratio of sine and cosine. So, you can essentially think of tangent as the slope of the line on a unit circle. Refer to the figure below. Another location along the unit circle where we get -1 is at 7pi/4, which is just 3pi/4 shifted by 180 degrees. Therefore, we can generalize this solution by adding 'npi' to 3pi/4 to get:

`x=3\pi /4+n\pi`

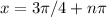
where 'n' is 0, 1, 2, 3...