Question

A 100 - foot fence is used to enclose a rectangular land plot. If the area of the land plot is 456 square feet, which equation can be used to determine the length (x) of the land plot?

Answer 1

`x^2-50x+456=0`

Explanation:

GIVEN: A
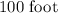`100\text{ foot}` fence is used to enclose a rectangular land plot. If the area of the land plot is
`456\text{ feet}^2`.

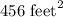TO FIND: equation to determine the length
`x` of the land plot.

SOLUTION:

total length of fence
`=100\text{ foot}`

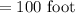
let the width of land plot be
`y`

perimeter of plot
`=2(x+y)=100`

`x+y=50`

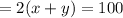
area of rectangular plot
`=\text{length}*\text{breadth}`

`xy=456`

`y=(456)/(x)`

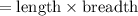
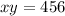
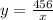
putting value of
`y`

`x+(456)/(x)=50`

`x^2-50x+456=0`

Hence required equation to get length
`x` of land plot is
`x^2-50x+456=0`

on solving

`x=38,12`

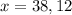
possible length of land plot is
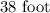`38\text{ foot}` and
`12\text{ foot}`