Question

What is the vertex of the quadratic function f(x) = (x - 6) (2x + 2)?

Answer 1

the coordinates of the vertex are: (2.5, -24.5)

Explanation:

Recall that when we have a quadratic in its standard form:

`f(x)=ax^2+bx+c`

the position of the x-coordinate for the vertex can be obtained via:

`x_(vertex)=(-b)/(2a)`

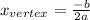
Then in order to find the vertex, first we write the expression in standard form:

`f(x)=(x-6)(2x+2)\\f(x)=2x^2+2x-12x-12\\f(x)=2x^2-10x-12`

Now that we have the values for the parameters "
`a`" and "b" we find the x of the vertex:

`x_(vertex)=(-b)/(2a)=(10)/(2*2)=(5)/(2)`

Now we use this x-value in the function to find the correspondent y-value of the vertex:

`f(x)=2x^2-10x-12\\f((5)/(2) )=2\,((5)/(2) )^2-10((5)/(2) )-12\\f((5)/(2) )=-24.5`

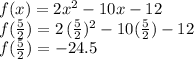
Then, the coordinates of the vertex are: (2.5, -24.5)