Question

The probability that an international flight leaving the United States is delayed in departing (event D) is .25. The probability that an international flight leaving the United States is a transpacific flight (event P) is .57. The probability that an international flight leaving the U.S. is a transpacific flight and is delayed in departing is .12.What is the probability that an international flight leaving the US is delayed in departing given that the flight is a transpacific flight? (Round answers to 4 decimal places)

Answer 1

Probability that an international flight leaving the US is delayed in departing given that the flight is a transpacific flight is 0.2105.

Explanation:

We are given that the probability that an international flight leaving the United States is delayed in departing (event D) is 0.25. The probability that an international flight leaving the United States is a transpacific flight (event P) is 0.57. The probability that an international flight leaving the U.S. is a transpacific flight and is delayed in departing is 0.12.

Let the probability that an international flight leaving the United States is delayed in departing = P(D) = 0.25

Probability that an international flight leaving the United States is a transpacific flight = P(P) = 0.57

Probability that an international flight leaving the U.S. is a transpacific flight and is delayed in departing =
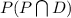`P(P \bigcap D)` = 0.12

Now, the probability that an international flight leaving the US is delayed in departing given that the flight is a transpacific flight is given by = P(D/P)

As we know that the conditional probability is given by;

P(A/B) =
`(P(A \bigcap B))/(P(B))`

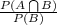
Similarly, P(D/P) =
`(P(D \bigcap P))/(P(P))`

=
`(0.12)/(0.57)`

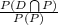
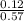
= 0.2105

Hence, the required conditional probability is 0.2105.