Question

In a sample of 6060 stores of a certain​ company, 5050 violated a scanner accuracy standard. It has been demonstrated that the conditions for a valid​ large-sample confidence interval for the true proportion of the stores that violate the standard were not met. Determine the number of stores that must be sampled in order to estimate the true proportion to within 0.050.05 with 9595​% confidence using the​ large-sample method.

Answer 1

Answer: 385

Explanation:

Given : In a sample of 60 stores of a certain​ company, 50 violated a scanner accuracy standard.

Let p be the true proportion of the stores that violate the standard.

Since , it has been demonstrated that the conditions for a valid​ large-sample confidence interval for p were not met.

So , to get accurate result , we take
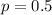`p=0.5` (It will give the minimum required sample size when prior information about p is not given )

Formula to find sample size :
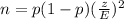`n=p(1-p)((z)/(E))^2`, where z= critical z-value for confidence interval and E is the margin of error

Since , E=0.05 and Critical z-value for 95​% confidence=1.96

`\Righatrrow\ n= (0.5)(0.5)((1.96)/(0.05))^2=384.16\approx385`

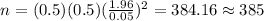
Hence, the number of stores that must be sampled= 385.