Answer:
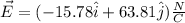
Step-by-step explanation:
In this case we have to work with vectors. Firs of all we have to compute the angles between x axis and the r vector (which points the charges):

the electric field has two components Ex and Ey. By considering the sign of the charges we obtain that:
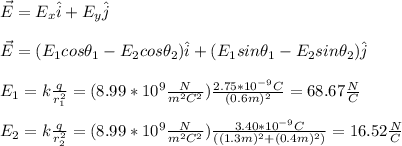
Hence, by replacing E1 and E2 we obtain:
![\vec{E}=[(68.67N/C)cos(90\°)-(16.52N/C)cos(17.10\°)]\hat{i}+[(68.67N/C)sin(90\°)-(16.52N/C)sin(17.10\°)]\hat{j}\\\\\vec{E}=(-15.78\hat{i}+63.81\hat{j})(N)/(C)](https://img.qammunity.org/2021/formulas/physics/college/ncefnw82mw4lr8epx8b0xjteoc2d779gi6.png)
hope this helps!!