Question

What is measured by the numerator of the z-score test statistic?​ a. ​The actual distance between M and µ ​b. Whether or not there is a significant difference between M and µ ​c. The position of the sample mean relative to the critical region ​d. The average distance between M and µ that would be expected if H0 was true

Answer 1

a. ​The actual distance between M and µ

Explanation:

The z-score test is a statistical test for which the distribution of the test is normally distributed under null hypothesis. It is used to test the mean for a known standard deviation. The z score (z) is the distance from the sample mean to the population mean in units of the standard error and is given by the equation:

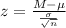`z=(M-\mu)/((\sigma)/(√(n) ) )`, where μ is the mean, ο is the standard deviation, n is the number of samples in the population and
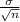`(\sigma)/(√(n) )` is the standard error.

The numerator of the z score is the distance from the sample mean (μ) to the population mean (M).