Answer:
Option C) Subtract 72° from 180°
Explanation:
We are given the following in the question:
Measure of exterior angle = 72°
Properties of exterior angles:
- An exterior angle of a polygon is an angle at a vertex of the polygon but outside the polygon.
- It is formed by one side and the extension of an adjacent side.
- The interior and exterior angles are supplementary.
- Thus, the sum of the interior angle and the exterior angle is 180 degrees.
- All the exterior angles of a regular polygon are equal in measure.
Thus, we can write:
Let x degrees be the measure of the interior angle. Thus, we can write:
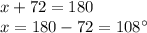
The measure of the interior angle is 108 degrees.
Thus, the correct answer is:
Option C) Subtract 72° from 180°