Question

Find the average rate of change of f(x) = 1x + 6 on [4,9). Round your answer to the nearest hundredth.

О 0.14

ООО

-0.14

Answer 1

The average rate of change of f(x) = x + 6 on [4,9) is 1.

Explanation:

Given a function y, the average rate of change S of
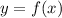`y=f(x)` in an interval
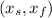`(x_(s), x_(f))` will be given by the following equation:

`S = (f(x_(f)) - f(x_(s)))/(x_(f) - x_(s))`

In this problem, we have that:

`f(x) = x + 6`

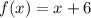
Interval [4,9]

Continuous function(no denominator or even root), this is why i can consider 9 a part of the interval for the calculation. So

`x_(s) = 4, x_(f) = 9, f(x_(s)) = f(4) = 10, f(x_(f)}) = f(9) = 15`.

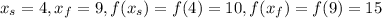So

`S = (f(x_(f)) - f(x_(s)))/(x_(f) - x_(s)) = (15 - 10)/(9 - 4) = 1`

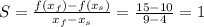
The average rate of change of f(x) = x + 6 on [4,9) is 1.