Question

Which ordered pairs are in a proportional relationship with (0.2, 0.3)?

A

(1.2, 2.3)

B

(2.7. 4.3)

C

(3.2, 4.8)

D

(3.5, 5.3)

E

(5.2, 7.8)

Answer 1

C and E

Explanation:

We are given that

`x_1=0.2,y_1=0.3`

`(y)/(x)=(0.3)/(0.2)=(3)/(2)`

`k=(y)/(x)=(3)/(2)`

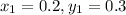
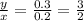
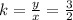
A.
`x_2=1.2,y_2=2.3`

`(y_2)/(x_2)=(2.3)/(1.2)=(23)/(12)\\eq=(3)/(2)`

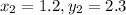
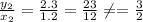
Hence, it is not in proportional relationship with (0.2,0.3)

B.(2.7,4.3)

`x_3=2.7,y_3=4.3`

`(y_3)/(x_3)=(4.3)/(2.7)=(43)/(27)\\eq(3)/(2)`

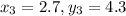
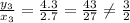
Hence, it is not in proportional relationship with (0.2,0.3).

C.(3.2,4.8)

`x_4=3.2,y_4=4.8`

`(y_4)/(x_4)=(4.8)/(3.2)=(3)/(2)`

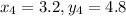
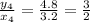
Hence, the ordered pair (3.2,4.8) are in a proportional relationship with (0.2,0.3).

D.(3.5,5.3)

`(y_5)/(x_5)=(5.3)/(3.5)=(53)/(35)\\eq (3)/(2)`

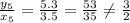
Hence, the ordered pair (3.5,5.3) are not in a proportional relationship with (0.2,0.3).

E.(5.2,7.8)

`(y_6)/(x_6)=(7.8)/(5.2)=(3)/(2)`

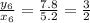
Hence, the ordered pair (5.2,7.8) are in a proportional relationship with (0.2,0.3).