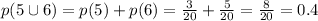Question

A number cube with faces labeled from 1 to 6 was rolled 20 times. Each time the number

cube was rolled, the number showing on the top face was recorded. The table shows the
results.
Results
Number Showing
on Top Face
Frequency
33
Based on these results, what is the experimental probability that the next time the number
cube is rolled it will land with 5 or 6 showing on the top face?

Answer 1

0.4

Explanation:

These are the relative frequencies of each face (data are missing in the text of the problem):

Number Showing on Top Face Frequency

1 0

2 3

3 3

4 6

5 3

6 5

The probability to obtain a certain number when throwing the dice is given by

`p(x_i)=(f_i)/(\sum f)`

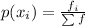
where

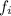`f_i` is the relative frequency of the number
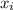`x_i` to occur

`\sum f` is the sum of the relative frequencies

Here the sum of the frequencies is:

`\sum f=0+3+3+6+3+5=20`

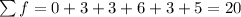
For number 5 here, we have:

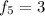`f_5 = 3` (from the table)

So the probability of getting a 5 is

`p(5)=(3)/(20)`

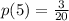
For number 6 here, we have

`f_6=5`

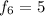
So the probability of getting a 6 is

`p(6)=(5)/(20)`

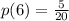
So the probability to obtain either a 5 or a 6 in the next rolling is:

`p(5\cup 6)=p(5)+p(6)=(3)/(20)+(5)/(20)=(8)/(20)=0.4`