Question

a liquid reactant is pumped through a horizontal, cylindrical, catalytic bed. The catalyst particles are spherical, 2mm in diameter. Data for two tests are available. For a flow rate of 12 ft3/hr the pressure drop is 9.6 psi; for 24 ft3/hr, the pressure drop is 24.1psi. The pump capacity produces a pressure drop of up to 50 psi. Requirements: what is the upper limit on the flow rate

Answer 1

The upper limit on the flow rate = 39.46 ft³/hr

Step-by-step explanation:

Using Ergun Equation to calculate the pressure drop across packed bed;

we have:

`(\delta P)/(L)= (150 \mu_oU(1- \epsilon )^2)/(d^2p \epsilon^3) + (1.75 \rho U^2(1-\epsilon))/(dp \epsilon^3)`

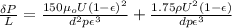
where;

L = length of the bed

`\mu` = viscosity

U = superficial velocity

`\epsilon` = void fraction

dp = equivalent spherical diameter of bed material (m)

`\rho` = liquid density (kg/m³)

However, since U ∝ Q and all parameters are constant ; we can write our equation to be :

ΔP = AQ + BQ²

where;

ΔP = pressure drop

Q = flow rate

Given that:

9.6 = A12 + B12²

Then

12A + 144B = 9.6 -------------- equation (1)

24A + 576B = 24.1 --------------- equation (2)

Using elimination methos; from equation (1); we first multiply it by 2 and then subtract it from equation 2 afterwards ; So

288 B = 4.9

B = 0.017014

From equation (1)

12A + 144B = 9.6

12A + 144(0.017014) = 9.6

12 A = 9.6 - 144(0.017014)

`A = (9.6 -144(0.017014)/(12)`

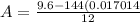
A = 0.5958

Thus;

ΔP = AQ + BQ²

Given that ΔP = 50 psi

Then

50 = 0.5958 Q + 0.017014 Q²

Dividing by the smallest value and then rearranging to a form of quadratic equation; we have;

Q² + 35.02Q - 2938.8 = 0

Solving the quadratic equation and taking consideration of the positive value for the upper limit of the flow rate ;

Q = 39.46 ft³/hr