Question

How many cubes with side lengths of \dfrac13\text{ cm} 3 1 ​ cmstart fraction, 1, divided by, 3, end fraction, start text, space, c, m, end text does it take to fill the prism? \dfrac{4}{3}\text{ cm} 3 4 ​ cm\dfrac{5}{3}\text{ cm} 3 5 ​ cm2\text{ cm}2 cm

Answer 1

120

Explanation:

Answer 2

81 cubes are needed to fill the given prism

Explanation:

Given that cubes with side lengths of
`(1)/(3)` cm

To find how many cubes needed to fill the prism of volume 3 cubic units:

Given, Volume of prism = 3 cubic units

The formula for the volume of the cube is

`V=a^3` ( ∵ side length=a)

`V =(1)/(3)* (1)/(3)* \fra{1}{3}`

`V=(1)/(27)` cubic units

∴ V= 0.0370 cubic units

To find the number of cubes needed to fill the prism, That is to divide the volume of cube by volume of the prism.

`(Volume of prism)/(Volume of cube)`

`=(3)/(0.0370)`

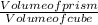
=81.081

= 81

∴ 81 cubes are needed to fill the given prism