Answer:
At E=204, the population increases by 3.2 thousand people for each billion dollar increase in the state's economy.
Explanation:
Given : A population researcher has found that the population P (in thousands) of a city in a particular year is a function of the number N (in thousands) of the jobs available in that year which is, in turn, a function of the economy E (in billions of dollars) of the state in which that city is located.
To find : The value of
 ?
?
Solution :
The researcher has determined that for a particular year,
E=204,
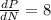 , and
, and
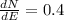 .
.
Using chain rule,
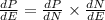
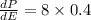
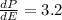
At E=204, the population increases by 3.2 thousand people for each billion dollar increase in the state's economy.