Answer:
3 cm
Explanation:
Formula
Volume of a cube =
 (where a is the side length)
(where a is the side length)
Given that the volume of the cube is 27 cm³, to find the side length, substitute the given volume into the equation and solve for
 :
:
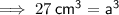
Cube root both sides:
![\implies \sqrt[3]{27\: \sf cm^3} =\sqrt[3]{a^3}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/wc7crpxrcmao5qz5gefb.png)
![\implies \sqrt[3]{27}\sqrt[3]{\sf cm^3} =\sqrt[3]{a^3}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/5udcaaggjsxybtenc9vs.png)
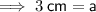
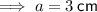
Therefore, the length of each side of the cube is 3 cm.