Question

A cylindrical bar of metal having a diameter of 20.9 mm and a length of 203 mm is deformed elastically in tension with a force of 46600 N. Given that the elastic modulus and Poisson's ratio of the metal are 64.4 GPa and 0.33, respectively, determine the following: (a) The amount by which this specimen will elongate in the direction of the applied stress. (b) The change in diameter of the specimen. Indicate an increase in diameter with a positive number and a decrease with a negative number.

Answer 1

`\delta l` = 0.428 mm

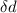`\delta d` = −0.0145 mm

Step-by-step explanation:

given data

diameter = 20.9 mm

length = 203 mm

force = 46600 N

elastic modulus = 64.4 GPa

Poisson's ratio = 0.33

solution

we get here first area of cross section that is

Area =
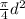`(\pi )/(4)d^2` .............1

put here value and we will get

Area =
`(\pi )/(4)* 20.9^2`

Area = 343.06 mm²

and

now we get here change in direction of applied stress that is

`\delta l` =
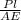`(Pl)/(AE)` .....................2

put here value and we will get

`\delta l` =
`(46600* 203)/(343.06* 64.4 * 10^3)`

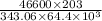
`\delta l` = 0.428 mm

and

we know Poisson ratio will be here express as

m =
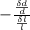`- ( (\delta d)/(d))/( (\delta l)/(l))` ........................3

0.33 =
`- ( (\delta d)/(20.9))/( (0.428)/(203))`

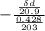
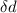`\delta d` = −0.0145 mm

so here change in diameter have -ve sign so diameter is decrease.