Question

write and simplify an expression for the surface area of a square pyramid with a base edge length of s in. & triangular faces with a height of 7 in. What is the surface area if the base edge length is 2.5 in.?

Answer 1

41.25

Explanation:

Answer 2

`A=14s+s^2`

`A=41.25\ in^2`

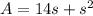
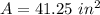
Explanation:

-Let s be the base lengths and h be the slant heights of the trinagles.

-Surface area of the pyramid is the sum of the areas of all of it's face and can be given as:

`Area=Area \ of \ Triangles +Base \ Area\\\\=4((1)/(2)sh)+s^2\\\\=2sh+s^2\\\\=s(2h+s)`

`h=7\\\\\therefore A=s(2h+s)\\\\=s(2* 7+s)\\\\=s(14+s)\\\\=14s+s^2`

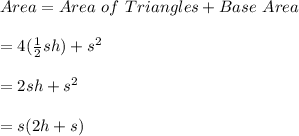
#We substitute the length and height dimensions in our simplified formula to calculate the Surface area as:

`A=s(2h+s)\\\\=2.5(2* 7+2.5)\\\\=41.25\ in^2`

Hence, the pyramid's surface area is
`41.25\ in^2`