Question

A 1,650-kg car stands on a square platform, and a 75-kg man simply stands on another square platform. These platforms are in fact two caps of a large oil-filled container. What must be the relation between the areas of the two platforms (that under the car and that under the man), for the man to be able to barely lift the car just by standing on his platform, without applying additional forces with his muscles?

Answer 1

It is concluded that the area of ​​the platform where the car is located must be 22 times greater than the area of ​​the platform where the man is located.

Step-by-step explanation:

The pressure on each platform is equal between them, then:

`(W_(1) )/(A_(1) ) =(W_(2) )/(A_(2) ) \\A_(1) =A_(2) (W_(1) )/(W_(2) )`

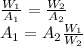
Where

m₁ = 1650 kg

m₂ = 75 kg

And:

`W_(1) =m_(1) *g=9.8*m_(1) \\W_(2) =m_(2) *g=9.8*m_(2)`

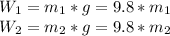
Replacing:

`A_(1) =A_(2) *((9.8*m_(1) )/(9.8*m_(2) ) )=A_(2)*((m_(1) )/(m_(2) ) )\\A_(1)=A_(2)*((1650)/(75) )=22A_(2)`

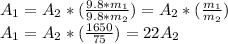
It is concluded that the area of ​​the platform where the car is located must be 22 times greater than the area of ​​the platform where the man is located.