Question

A weight of 50.0 N is suspended from a spring that has a force constant of 210 N/m. The system is undamped and is subjected to a harmonic driving force of frequency 11.5 Hz, resulting in a forced-motion amplitude of 4.00 cm. Determine the maximum value of the driving force.

Answer 1

The maximum value of the driving force is 1044.01 N.

Step-by-step explanation:

Given that,

Weight of the object, W = 50 N

Force constant of the spring, k = 210 N/m

The system is undamped and is subjected to a harmonic driving force of frequency 11.5 Hz.

Amplitude, A = 4 cm

We need to find the maximum value of the driving force. The force is given by the product of mass and maximum acceleration as :

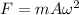`F=mA\omega^2` .....(1)

A is amplitude

m is mass,

`m=(W)/(g)\\\\m=(50)/(10)\\\\m=5\ kg`

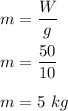
`\omega` is angular frequency

Angular frequency is given by :

`\omega=2\pi f\\\\\omega=2\pi * 11.5\\\\\omega=72.25\ rad/s`

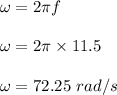
Equation (1) becomes :

`F=5* 0.04* 72.25^2\\\\F=1044.01\ N`

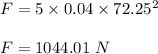
So, the maximum value of the driving force is 1044.01 N.