Question

A multiple choice test is composed of 10 questions. Each question has four possible answers, only one of the four possible answers is correct. A student randomly selects the answer for each question. Assume that all selections are independent. Let X count the student's number of correct answers on the test. a) What is the expected number of correct answers? Round your answer to the nearest tenth. b) What is the standard deviation of the number of correct answers? Round your answer to the nearest hundredth. c) What values of the random variable are within two standard deviations of the mean? d) What is the probability that the student answers exactly 4 questions correctly? Round your answer to the nearest ten- thousandth. e) If at least 6 questions must be answered correctly to pass the test, what is the probability that the student passes the test? Round your answer to the nearest ten-thousandth.

Answer 1

a)2.5

b) 1.37

c) -0.24, 5.24

d) 0.1460

e) 0.0420

Explanation:

Given:

n = 10

P = 1/4 = 0.25

a) expected number of answers

= n * p

= 10 * 0.25 = 2.5

b) standard deviation

`= √(np(1-p))`

`= √(10*0.25*(1-0.25))`

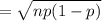
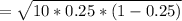
= 1.37

c)• u-26 = 2.5 - 2(1.37)

= 2.5 - 2.75

= -0.24

• u+26= 2.5 + 2(1.37)

= 5.24

d) Pobability that the student answers exactly 4 questions correctly

`= P(x=4) = [10, 4] (0.25)^4 (0.75)^6`

= 0.1460

e) P(x≥6)=P(x=6)+P(x=7)+P(x=8)+P(x=9)+P(x=10)

`= [10 ,6] (0.25)^6(0.75)^4+ [10, 7](0.25)^7(0.75)^3 + [10, 8](0.24)^8(0.75)^2 + [10, 9] (0.25)^9(0.75)^2 + [10, 10] (0.25)^10(0.75)^0`

= 0.03845+0.00309+0.00039+0.0000286+0.000000954

= 0.0420

(Part (e) is also attached for easier understanding)