Question

A chemist examines 15 geological samples for potassium chloride concentration. The mean potassium chloride concentration for the sample data is 0.376 cc/cubic meter with a standard deviation of 0.0012. Determine the 95% confidence interval for the population mean potassium chloride concentration. Assume the population is approximately normal.

Required:
Find the critical value that should be used in constructing the confidence interval. Round your answer to three decimal places.

Answer 1

95% Confidence interval: (0.375,0.377)

Explanation:

We are given the following in the question:

Sample mean,
`\bar{x}` = 0.376 cc/cubic meter

Sample size, n = 15

Alpha, α = 0.05

Sample standard deviation, s = 0.0012

Degree of freedom =

`=n-1\\=15-1\\=14`

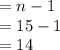
95% Confidence interval:

`\bar{x} \pm t_(critical)\displaystyle(s)/(√(n))`

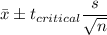
Putting the values, we get,

`t_(critical)\text{ at degree of freedom 14 and}~\alpha_(0.05) = \pm 2.145`

`0.376 \pm 2.145((0.0012)/(√(15)) )\\\\ = 0.376 \pm 0.0006\\\\ = (0.3754,0.3766)\approx (0.375,0.377)`

(0.375,0.377) is the required 95% confidence interval for the population mean potassium chloride concentration.