Question

Two identical cars, one on the moon and one on the earth, have the same speed and are rounding banked turns that have the same radius r. There are two forces acting on each car, its weight mg and the normal force FN exerted by the road. Recall, that the weight of an object on the moon is about one sixth of its weight on earth. How does the centripetal force on the moon compare with that on earth

Answer 1

The value of the centripetal forces are same.

Step-by-step explanation:

Given:

The masses of the cars are same. The radii of the banked paths are same. The weight of an object on the moon is about one sixth of its weight on earth.

The expression for centripetal force is given by,

`F_(c) = (mv^(2))/(r)`

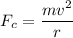
where,
`m` is the mass of the object,
`v` is the velocity of the object and
`r` is the radius of the path.

The value of the centripetal force depends on the mass of the object, not on its weight.

As both on moon and earth the velocity of the cars and the radii of the paths are same, so the centripetal forces are the same.