Answer:
(a). Index of refraction are
 = 1.344 &
= 1.344 &
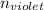 = 1.406
= 1.406
(b). The velocity of red light in the glass
 2.23 ×
2.23 ×
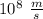
The velocity of violet light in the glass
 2.13 ×
2.13 ×
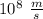
Step-by-step explanation:
We know that
Law of reflection is

Here
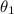 = angle of incidence
= angle of incidence
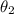 = angle of refraction
= angle of refraction
(a). For red light
1 ×
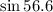 =
=
 ×
×
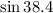
 = 1.344
= 1.344
For violet light
1 ×
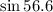 =
=
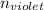 ×
×
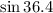
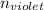 = 1.406
= 1.406
(b). Index of refraction is given by
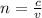
 = 1.344
= 1.344
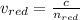
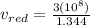
 2.23 ×
2.23 ×
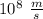
This is the velocity of red light in the glass.
The velocity of violet light in the glass is given by
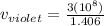
 2.13 ×
2.13 ×
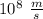
This is the velocity of violet light in the glass.