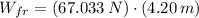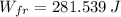Question

A factory worker pushes a 31.0 kg crate a distance of 4.20 m along a level floor by pushing downward at an angle of 32.0 ∘ below the horizontal. The coefficient of kinetic friction between the crate and floor is 0.260.

Now assume that, instead of the previous situation, the worker pushes harder (without changing the direction of his push), so that the crate moves with increasing speed. Assume the crate is pushed over the same displacement as before.

How much work is done on the crate by the force of friction during the displacement of 4.20 m when the pushing force is 118.00 N?

Answer 1

`W_(fr) = 281.539\,J`

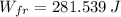
Step-by-step explanation:

The kinetic force of friction is:

`f = (0.260)\cdot (31\,kg) \cdot (9.807\,(m)/(s^(2)))\cdot \cos 32^(\textdegree)`

`f = 67.033\,N`

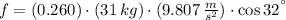
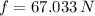
Before calculating work, it is require to determine if force exerted on the crate is enough to move it at least. The equation of equilibrium for the crate is:

`\Sigma F = F - f + m\cdot g \cdot \sin \theta = m \cdot a`

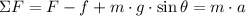
The acceleration experimented by the crate is:

`a = (F-f)/(m) + g\cdot \sin \theta`

`a = (118\,N-67.033\,N)/(31\,kg)+(9.807\,(kg)/(m^(2)) )\cdot \sin 32^(\textdegree)`

`a = 6.841\,(m)/(s^(2))`

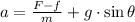
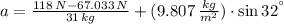
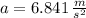
This positive result indicates that motion is physically reasonable. Hence, the work done by the force of friction is:

`W_(fr) = (67.033\,N)\cdot (4.20\,m)`

`W_(fr) = 281.539\,J`