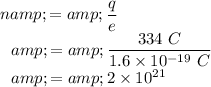Question

Two identical 9.10-g metal spheres (small enough to be treated as particles) are hung from separate 700-mm strings attached to the same nail in a ceiling. Surplus electrons are added to each sphere, and then the spheres are brought in contact with each other and released. Their equilibrium position is such that each string makes a 17.0° angle with the vertical.

How many surplus electrons are on each sphere?

Answer 1

The number of electrons surplussed on each surface is
`\bf{2 * 10^(21)}`.

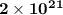Step-by-step explanation:

Given:

The masses of the sphere,
`m = 9.10~g`

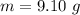
The length of the strings,
`L = 700~mm`

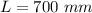
The angle made by each string with vertical,
`\theta = 17.0^(0)`

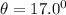
According to the diagram, the equilibrium condition for the vertical components of the forces acting on each sphere can be written as

`T \cos \theta = mg~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(1)`

The equilibrium condition for the horizontal components of the forces acting on each sphere can be written as

`T \sin \theta = F~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(2)`

Here,
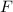`F` is the electrostatic force experienced by the metal spheres.

The value of the electrostatic force is given by

`F = (q^(2))/(r^(2))~~~~~~~~~~~~~~~~~~~~~~~~~(3)`

Here,
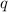`q` is the charge on each sphere and
`r` is the distance between them.

Referring to the figure, from
`\bigtriangleup QOR`,

`&& (r/2)/(L) = \sin \theta\\&or,& r = 2L \sin \theta`

Substituting the value of
`r` in equation (3), we have

`F = (q^(2))/((2L \sin \theta)^(2))~~~~~~~~~~~~~~(4)`

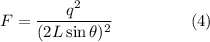
From equation (1),

`T &=& (mg)/(\cos \theta)\\&=& ((9.10~g)(980~cm/s^(2)))/(\cos 17^(0))\\&=& 9325.7~dyn`

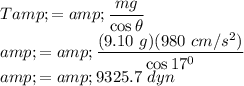
Substituting the values of
`T` and
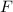`F` in equation (2), we have

`&& (9325.7~dyn) \sin 17^(0) = (q^(2))/((2 * 70 * \sin 17^(0)))\\&or,& q^(2) = ((9325.7~dyn) \sin 17^(0))(2 * 70 * \sin 17^(0))\\&or,& q = 334~C`

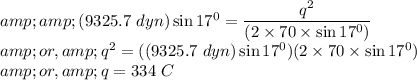
If
`n` is the number if electron surplussed with the metal sphere, then

`ne = q~~~~~~~~~~~~~~~~~~~~~~~~~~(5)`

Here,
`e` is the electronic charge.

Substituting the value of
`e` and
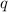`q` in equation (5), we have

`n &=& (q)/(e)\\~~~&=& (334~C)/(1.6 * 10^(-19)~C)\\~~~&=& 2 * 10^(21)`