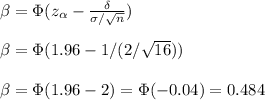Question

he mean water temperature downstream from a discharge pipe at a power plant cooling tower is specified to be 99 degrees F. Past experience shows that the population standard deviation is 2 degrees F. The water temperature is taken on 16 random days and the sample mean computed to be 98 degrees F. If the true population mean water temperature is 100 degrees F, what is the probability that we fail to reject H0 when H0 is false

Answer 1

The probability that we fail to reject H0 when H0 is false is P=0.48.

Explanation:

Failing to reject the null hypothesis when it is false is a Type II error.

The probability of a Type II error is written as β.

We have a null hypothesis that states that the mean is 99 degrees F. The standard deviation of the population is known and is 2 degrees F.

We assume a significance level of 0.05 and a two-tails test.

The z-value for this level of significance is z=1.96.

The value of β can be calculated as:

`\beta=\Phi(z_(\alpha)-(\delta)/(\sigma/√(n)))`

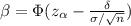
Where Φ is the standarized normal function and δ is the difference between the real mean and the null hypothesis mean.

Then, δ is:

`\delta=\mu-\mu_0=100-99=1`

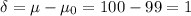
Now, we can calculate β as:

`\beta=\Phi(z_(\alpha)-(\delta)/(\sigma/√(n)))\\\\\beta=\Phi(1.96-1/(2/√(16)))\\\\\beta=\Phi(1.96-2)=\Phi(-0.04)=0.484`