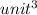Answer:
32
Explanation:
Given:
- The slant length 10 units
- A right square pyramid with base edges of length 8

Now we use Pythagoras to get the slant height in the middle of each triangle:
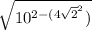 =
=
 =
=
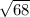 units
units
One again, you can use Pythagoras again to get the perpendicular height of the entire pyramid.
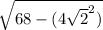 =
=
 = 6 units.
= 6 units.
Because slant edges of length 10 units each is cut by a plane that is parallel to its base and 3 units above its base. So we have the other dementions of the small right square pyramid:
- The height 3 units
- A right square pyramid with base edges of length 4

So the volume of it is:
V = 1/3 *3* 4

= 32