Answer with Step-by-step explanation:
We are given that
C(x)=
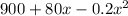
a.x=150
Substitute the value of x
Total cost of 150 appliances=
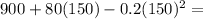 $8400
$8400
Average cost per appliance=
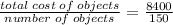 =$56
=$56
b.Differentiate w.r.t x
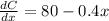
Substitute x=150
Marginal cost when 150 appliances are produced
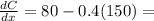 $20
$20
c.Cost of 151 appliances=
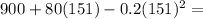 $8419.8
$8419.8
Cost of 151th appliance=Cost of 151 appliances-Cost of 150 appliances
Cost of 151th appliance=$8419.8-$8400=
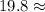 $20
$20
Hence, the marginal cost when 150 appliances are produced is approximately equal to the cost of producing one more appliance after the first 150 have been made .