Question

Suppose that the probabilities that an answer can be found on Google is .95, on Answers is .92, and on both Web sites is .874. Are the possibilities of finding the answer on the two Web sites independent? a) Yes, because (.95)(.92) = .874 b) No, because (.95)(.92) = .874 c) Yes, because .95 > .92 > .874 d) No, because .5(.95+.92) ≠ .874 e) Cannot be answered with this information

Answer 1

The correct option is

a) Yes, because (0.95)(0.92) = 0.874

Explanation:

An event is independent if the following condition is satisfied

`P(A\bigcap B)= P(A)P(B)`

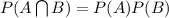
Therefore, since the the probability that the answer can be found in Google = 0.95 and the probability that the answer can be found in Answers = 0.92

The probability that the answer can be found on both websites,

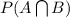`P(A\bigcap B)` = 0.874

We check, P(A) × P(B) = 0.95×0.92 = 0.874 =
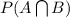`P(A\bigcap B)` = 0.874

Therefore, the possibilities of finding the event on the two websites is independent.