Answer:
690 units
Step-by-step explanation:
daily demand = 60 units
standard deviation = 10 units
review period = 10 days
lead time = 2 days
stock = 100 units
service probability = 98%
order quantity using fix time period formula:

- standard deviation =
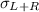 = √(10 + 2) x 10 = 34.64
= √(10 + 2) x 10 = 34.64 - z for 98% = 2.05
- I = stock = 100
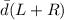 = 60 x (10 + 2)
= 60 x (10 + 2)
order quantity = (60 x 12) + (2.05 x 34.64) - 100 = 690 units