Question

A cable with mass 0.5 kilograms per meter (kg/m) is used to lift 150 kg of coal up a mine shaft 50 meters deep. Set up the integral that will calculate the work needed to lift the load one quarter of the way up the shaft. (Set up the integral only, no need to compute its value.)

Answer 1

`W=-\int_(0)^(50)[150-0.5y]dy`

Step-by-step explanation:

the integral for the work is:

`W=\int\vec{F}\cdot d\vec{l}=\int(-M(y)g)dy`

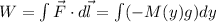
the work is against the gravitational force. While the coal is going up, M(y) is changing due to the length of the cable is lower. We can describe this by using the following formula

`M(y)=150kg-0.5(kg)/(m)y`

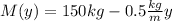
Thus , the integral for the work is:

`W=-\int_(0)^(50)[150-0.5y]dy`

hope this helps!!