Question

In a coffee‑cup calorimeter, 65.0 mL of 0.890 M H 2 SO 4 was added to 65.0 mL of 0.260 M NaOH . The reaction caused the temperature of the solution to rise from 23.78 ∘ C to 25.55 ∘ C. If the solution has the same density as water (1.00 g/mL) and specific heat as water (4.184 J/g‑K), what is Δ H for this reaction (per mole of H 2 O produced)? Assume that the total volume is the sum of the individual volumes.

Answer 1

Answer : The enthalpy of neutralization is, 56.96 kJ/mole

Explanation :

First we have to calculate the moles of H₂SO₄ and NaOH.

`\text{Moles of }H_2SO_4=\text{Concentration of }H_2SO_4* \text{Volume of solution}=0.890mole/L* 0.065L=0.0578mole`

`\text{Moles of NaOH}=\text{Concentration of NaOH}* \text{Volume of solution}=0.260mole/L* 0.065L=0.0169mole`

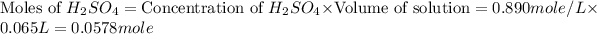
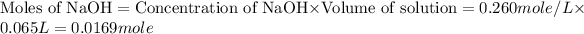
The balanced chemical reaction will be,

`H_2SO_4+2NaOH\rightarrow Na_2SO_4+2H_2O`

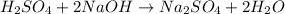
From the balanced reaction we conclude that,

As, 2 mole of NaOH neutralizes by 1 mole of H₂SO₄

So, 0.0169 mole of NaOH neutralizes by 0.00845 mole of H₂SO₄

That means, NaOH is a limiting reagent and H₂SO₄ is an excess reagent.

Now we have to calculate the moles of H₂O.

As, 2 mole of NaOH react to give 2 mole of H₂O

So, 0.0169 mole of NaOH react to give 0.0169 mole of H₂O

Now we have to calculate the mass of water.

As we know that the density of water is 1.00 g/ml. So, the mass of water will be:

The volume of water =
`65.0ml+65.0ml=130ml`

`\text{Mass of water}=\text{Density of water}* \text{Volume of water}=1.00g/ml* 130ml=130g`

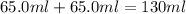
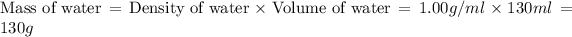
Now we have to calculate the heat absorbed during the reaction.

`q=m* c* (T_(final)-T_(initial))`

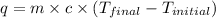
where,

q = heat absorbed = ?

`c` = specific heat of water =
`4.184J/g^oC`

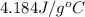
m = mass of water = 130 g

`T_(final)` = final temperature of water =
`23.78^oC`

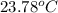
`T_(initial)` = initial temperature of metal =
`25.55^oC`

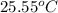
Now put all the given values in the above formula, we get:

`q=130g* 4.184J/g^oC* (25.55-23.78)^oC`

`q=962.7J`

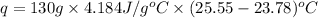
Thus, the heat released during the neutralization = -962.7 J

Now we have to calculate the enthalpy of neutralization.

`\Delta H=(q)/(n)`

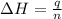
where,

`\Delta H` = enthalpy of neutralization = ?

q = heat released = -962.7 J

n = number of moles used in neutralization = 0.0169 mole

`\Delta H=(-962.7J)/(0.0169mole)=-56964.49J/mole=-56.96kJ/mol`

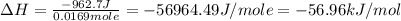
The negative sign indicate the heat released during the reaction.

Therefore, the enthalpy of neutralization is, 56.96 kJ/mole