Answer:
she will fall to an height of 21.09° only, beyond such an height ; the spring will break.
Katya will not be able to swing to the platform because the angle will be between -21.09° and 21.09° from vertical
Step-by-step explanation:
If the distance is from the point of angle A to angle B , partitioned by a perpendicular line in the middle then, The conservation of energy between A and B can be expressed as :
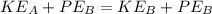
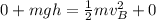
where ; the height h =
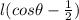
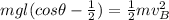
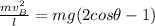
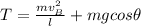
T = mg(3 cos θ - 1)
Given that:
T = 180 % = 1.8 mg
Then:
1.8 mg = mg(3 cos θ - 1)
2.8 mg = (3 cos θ - 1)
cos θ =
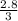
θ = cos ⁻¹ (0.933)
θ = 21.09°
Therefore, she will fall to an height of 21.09° only, beyond such an height ; the spring will break.
Katya will not be able to swing to the platform because the angle will be between -21.09° and 21.09° from vertical