Question

Two carts with masses of 12.5 kg and 3.5 kg, respectively, move in opposite directions on a frictionless horizontal track with speeds of 6.0 m/s and 1.0 m/s, respectively. The carts stick together after colliding head-on. Find the final speed of the two carts. m/s

Answer 1

Step-by-step explanation:

Parameters given:

Mass of first cart, m = 12.5 kg

Mass of second cart, M = 3.5 kg

Initial velocity of first cart, u = 6.0 m/s

Initial velocity of second cart, v = 1.0 m/s

NOTE: Both carts stick together after collision, hence, they have the same final velocity, v

Using the principle of conservation of momentum, we have that the total initial momentum of the system is equal to the final momentum of the system:

Total Initial Momentum = Total Final Momentum

mu + MU = (m + M)v

Making v subject of formula:

`v = (mu+MU)/(m + M)`

`v = ((12.5 * 6) + (3.5 * 1))/(12.5 + 3.5 ) \\\\\\v = (75 + 3.5)/(16) \\\\\\v = (78.5)/(16)\\ \\\\v = 4.91 m/s`

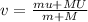
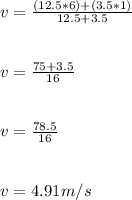
The final speed of the two carts is 4.91 m/s.