Answer:
80% probability that it will take Victoria greater than 8 minutes (total) for a puzzle that she has already been working on for 7 minutes
Explanation:
An uniform probability is a case of probability in which each outcome is equally as likely.
For this situation, we have a lower limit of the distribution that we call a and an upper limit that we call b.
The probability that we find a value X greater than x is given by the following formula.

Uniformly distributed random time between 4 minutes and 12 minutes.
This means that
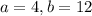
What is the probability that it will take Victoria greater than 8 minutes (total) for a puzzle that she has already been working on for 7 minutes?
Already working for 7 minutes, so a is updated to 7.
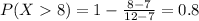
80% probability that it will take Victoria greater than 8 minutes (total) for a puzzle that she has already been working on for 7 minutes