Question

A true/false test consists of 25 questions. A student knows the correct answer to 10 of the questions. She guesses each of the other answers independently based on the toss of a coin. a) Find the expectation of the number of correct answers.

Answer 1

The expectation of the number of correct answers is 17.5

Explanation:

For each question, there are only two possible outcomes. Either the student guesses it correctly, or he does not. The probability of a student guessing the answer of a question correctly is independent of other questions. So we use the binomial probability distribution to solve this question.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

The expected value of the binomial distribution is:

`E(X) = np`

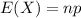
In this problem, we have that:

25 questions.

The student know the answer for 10 questions. So for these 10 questions, p = 1.

On the other 25-10 = 15, she will guess, which there is one correct answer out of 2 options(true/false), so p = 1/2 = 0.5. So

`E(X) = 10*1 + 15*0.5 = 17.5`

The expectation of the number of correct answers is 17.5