Question

Mary wants to hang a mirror in her room. The mirror and frame must have an area of 7 square feet. The mirror is 2 feet wide and 3 feet long. Which quadratic equation can be used to determine the thickness of the frame, x?

Answer 1

its D

Explanation:

got it rt on the test

Answer 2

The quadratic equation used to determine the thickness of frame is
`\left(3+2x\right)* \left(2+2x\right)=7`

Explanation:

Refer to the attachment for the diagram.

Consider EFGH as the the frame and ABCD as the mirror which is to be hanged.

Let me the "x" be the thickness of the frame as shown in diagram.

Given that, BC = 3 ft and DC = 2 ft which is the dimensions of the mirror.

Now to calculate the length and width of the frame consider following calculation,

Width of frame HG = x + DC + x

Width of frame HG = x + 2 + x

Width of frame HG = 2 + 2x

2x is the addition of the thickness which is present on both side of mirror

Length of frame FG = x + BC + x

Length of frame FG = x + 3 + x

Length of frame FG = 3 + 2x

Since it is an rectangular frame, so using the formula for area of rectangle

`Area\:of\:rectangle = length * width`

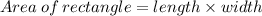
Given that area of frame is
`7\:ft^(2)`

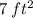
Substituting the value,

`7 = \left(3+2x\right)* \left(2+2x\right)`

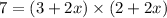
Therefore, the quadratic equation used to determine the thickness of frame is
`\left(3+2x\right)* \left(2+2x\right)=7`