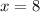Question

Find the equation of the axis of symmetry of the following parabola algebraically.

y=3x²-48x+177

Answer 1

X=8

Explanation:

Answer 2

the equation of the axis of symmetry is
`x=8`

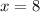
Explanation:

Recall that the equation of the axis of symmetry for a parabola with vertical branches like this one, is an equation of a vertical line that passes through the very vertex of the parabola and divides it into its two symmetric branches. Such vertical line would have therefore an expression of the form:
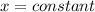`x=constant`, being that constant the very x-coordinate of the vertex.

So we use for that the fact that the x position of the vertex of a parabola of the general form:
`y=ax^2+bx+c`, is given by:

`x_(vertex)=(-b)/(2\,a)`

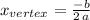
which in our case becomes:

`x_(vertex)=(-b)/(2\,a) \\x_(vertex)=(48)/(2\,(3)) \\x_(vertex)=(48)/(6) \\x_(vertex)=8`

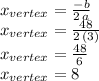
Then, the equation of the axis of symmetry for this parabola is:

`x=8`