Question

Assume that the speed of light in a vacuum has the hypothetical value of 18.0 m/s. A car is moving at a constant speed of 14.0 m/s along a straight road. A home owner sitting on his porch sees the car pass between two telephone poles in 4.12 s. How much time does the driver of the car measure for his trip between the poles

Answer 1

The time measured by the driver of the car measure for his trip between the poles is 2.58 seconds.

Step-by-step explanation:

Given that,

Let us speed of light in a vacuum has the hypothetical value, c = 18 m/s

A car is moving at a constant speed of 14.0 m/s along a straight road, v = 14 m/s

The observer's time interval is 4.12 s

We need to find the time measured by the driver of the car measure for his trip between the poles. Let it is t. The observer's time interval is given by :

`T=\frac{t}{\sqrt{1-(v^2)/(c^2)} }\\\\t=T* \sqrt{1-(v^2)/(c^2)} \\\\t=4.12* \sqrt{1-((14)^2)/((18)^2)} \\\\t=2.58\ s`

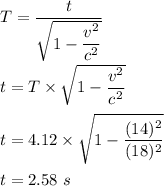
So, the time measured by the driver of the car measure for his trip between the poles is 2.58 seconds.