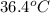Answer : The final temperature of the water is,
Explanation :
First we have to calculate the moles of benzene.
Mass of
 = 8.700 g
= 8.700 g
Molar mass of
 = 78 g/mol
= 78 g/mol
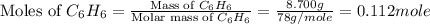
Now we have to calculate the heat produced by the reaction.
As, 2 moles of benzene burns to give heat = 6542 kJ
So, 0.112 moles of benzene burns to give heat =
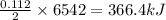
Now we have to calculate the final temperature of the water.
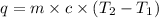
where,
q = heat produced = 366.4 kJ = 366400 J
m = mass of water = 5691 g
c = specific heat capacity of water =
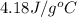
 = initial temperature =
= initial temperature =
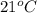
 = final temperature = ?
= final temperature = ?
Now put all the given values in the above formula, we get:
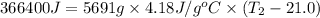
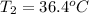
Thus, the final temperature of the water is,