Answer:
The maximum period of rotation of the cylinder is 2.84 seconds
Step-by-step explanation:
Radius of the cylinder, r = 5 m
Coefficient of static friction,
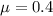
The frictional force,
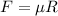
The normal reaction, R is a centripetal force:
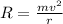
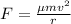
The force due to gravity, F = mg
For equilibrium, the force due to gravity must equal the frictional force
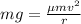
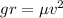
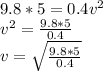
v = 11.07 m/s
The angular speed, w = v/r
w = 11.07/5
w = 2.214 rad/s
For a complete revolution through a circle, θ = 2π rad
w = θ/t
t = θ/w
t = 2π/2.214
t = 2.84 seconds