Answer:
Probability that a random sample of 50 U.S. adults has less than 35% with this opinion is 0.22965.
Explanation:
We are given that Research in March 2019 suggests that 40% of U.S. adults approve of way President Trump is running the country. We randomly sample 50 U.S. adults and find that 35% approve of way President Trump is running the country.
Let
 = sample proportion of U.S.adults politicians who approve of way President Trump is running the country.
= sample proportion of U.S.adults politicians who approve of way President Trump is running the country.
The z-score probability distribution for sample proportion is given by;
Z =
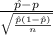 ~ N(0,1)
~ N(0,1)
where,
 = sample proportion
= sample proportion
p = population proportion = 40%
n = sample of U.S. adults = 50
The Z-score measures how many standard deviations the measure is away from the mean. After finding the Z-score, we look at the z-score table and find the p-value (area) associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X.
So, probability that a random sample of 50 U.S. adults has less than 35% with this opinion is given by = P(
 < 0.35)
< 0.35)
P(
 < 0.51) = P(
< 0.51) = P(
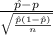 <
<
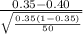 ) = P(Z < -0.74) = 1 - P(Z
) = P(Z < -0.74) = 1 - P(Z
 0.74)
0.74)
= 1 - 0.77035 = 0.22965
Now, in the z table the P(Z
 x) or P(Z < x) is given. So, the above probability is calculated by looking at the value of x = 0.74 in the z table which has an area of 0.77035.
x) or P(Z < x) is given. So, the above probability is calculated by looking at the value of x = 0.74 in the z table which has an area of 0.77035.
Therefore, probability that a random sample of 50 U.S. adults has less than 35% with this opinion is 0.22965.