Question

A student takes a part-time job to earn for summer travel. The number of hours, , the student has to work is inversely proportional to the wage, , in dollars per hour. (a) Write an expression for the function . Note that "" is already provided. Do not include this in your submitted response to this question. MathPAD Response msnViewer_res_c07q_eoc_1_18_mathpad_57_svg ViewEdit (b) How many hours does the student have to work if the job pays an hour

Answer 1

(a)
`h = (2400)/(w)`

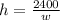
(b)300 Hours

(c)150 Hours

(d)Reduced and halved.

(e)
`w = (2400)/(h)`

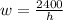
Explanation:

(a) The number of hours worked is inversely proportional to the wage.

This is written as:

`h \propto (1)/(w) \\\text{Introducing variation constant k}\\h = (k)/(w)\\$Since the money to be raised is constant, k = $ \$2400\\Therefore, h = (2400)/(w)`

(b)If the student earns $8 an hour

w=$8

`\text{Number of Hours required, h} = (2400)/(8) =300 Hours`

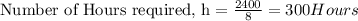
(c)When the wage per hour =$16

When w=$16

`\text{Number of Hours required, h} = (2400)/(16) =150 Hours`

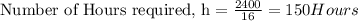
The number of hours reduced and is in fact halved.

(d)

`\text{When the wage per hour =w}, h = (2400)/(w)\\\text{When the wage per hour =2w}, h = (2400)/(2w)=(1)/(2) X (2400)/(w) =(h)/(2)`

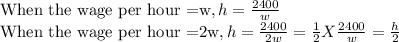
The effect of raising the wage from $w to $2w per hour is that the number of hours required to work is reduced and exactly halved.

(e)The wage per hour is inversely proportional to the number of hours.

In fact,

`\text{From h = }(2400)/(w)\\\text{Cross Multiplying}\\hw=2400\\\text{Dividing both sides by h}\\w = (2400)/(h)`