Question

An automobile manufacturer would like to know what proportion of its customers are dissatisfied with the service received from their local dealer. The customer relations department will survey a random sample of customers and compute a 95% confidence interval for the proportion that are dissatisfied. From past studies, they believe that this proportion will be about 0.28. Find the sample size needed if the margin of error of the confidence interval is to be no more than 0.02. (Round your answer up to the nearest whole number.)

Answer 1

The sample size needed if the margin of error of the confidence interval is to be no more than 0.02.

n= 2015

Explanation:

Given the customer relations department will survey a random sample of customers and compute a 95% confidence interval for the proportion that are dissatisfied

Given data from past studies, they believe that this proportion will be about 0.28

The proportion of success(p) = 0.28

We know that the margin of error of 95% of intervals of given proportion

margin of error =
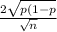`(2√(p(1-p) )/(√(n) )`…(i)

Given margin of error = 0.02

Substitute values in equation (i) cross multiplication √n

0.02 √n = 2√0.28X0.72

On calculation, we get √n = 44.89

squaring on both sides, we get

n = 2015

Conclusion:-

The sample size needed if the margin of error of the confidence interval is to be no more than 0.02.

n= 2015