Answer:
Hoop.
Step-by-step explanation:
The angular acceleration performed at a given torque:
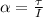
The moments of inertia of each element are described below:
Hoop
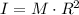
Solid sphere
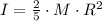
Flat disk
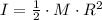
Hollow sphere
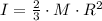
The greater the moment of inertia, the greater the torque to obtain the same angular acceleration. Therefore, the hoop requires the largest torque to receive the same angular acceleration.