Answer:
52 feet by 13 feet.
Step-by-step explanation:
Given:
The length of a rectangular pool is to be four times its width.
Sidewalk is 6 feet wide will surround the pool.
If a total area of 1600 square feet has been set aside for the area of the pool and the sidewalk.
Question asked:
What are the dimensions of the pool?
Solution:
Let width of a rectangular pool =

Then the length of a rectangular pool will be =
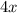
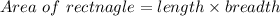

Combined length of pool and the sidewalk = length of pool + width of sidewalk + width of sidewalk
Combined length of pool and the sidewalk =
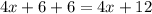
Similarly, Combined width of pool and the sidewalk =
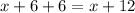
Combined area of pool and the sidewalk = 1600
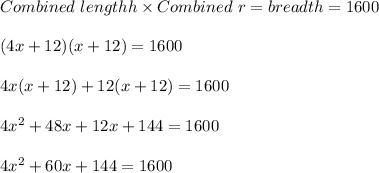
Subtracting both sides by 144

Since dimensions can never be in negative, hence
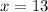
Width of a rectangular pool =
 = 13 feet
= 13 feet
The length of a rectangular pool will be =
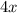 =
=
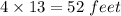
Therefore, the dimensions of the pool are 52 feet by 13 feet.