Question

an inlet pipe and a hose together can fill the pond in 10 hours. The inlet pipe alone can complete the job in one hour less time than the hose alone. Find the time that the hose can complete the job alone and the time that the inlet pipe can complete the job alone

Answer 1

The hose pipe can fill the pond in 21.465 hours.

The inlet pipe can fill the pond in 20.465 hours.

Explanation:

Given that, a inlet pipe and a hose pipe together can fill the pond in 10 hours.

The inlet pipe alone can fill the pond in one hour less time than the hose pipe can fill the pond.

Let the hose pipe can complete the job in x hours.

Then the inlet pipe can fill the pond in (x-1) hours.

The rate of filling of hose pipe is
`=(1)/(x)`

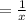
The rate of filling of inlet pipe is
`=(1)/(x-1)`

The rate of filling of both pipes is =
`\frac 1{10}`

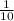
According to the problem,

`(1)/(x)+(1)/(x-1)=(1)/(10)`

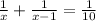
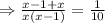`\Rightarrow (x-1+x)/(x(x-1))=\frac1{10}` [ simplifying the fraction]

`\Rightarrow (2x-1)/(x^2-x)=\frac1{10}`

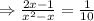
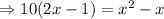`\Rightarrow 10(2x-1)= x^2-x` [ Multiplying 10(x²-x) both sides]

`\Rightarrow x^2-x= 20x-10`

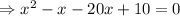`\Rightarrow x^2-x-20x+10=0` [ simplifying ]

`\Rightarrow x^2-21x+10=0`

`\Rightarrow x=(-(-21)\pm√((-21)^2-4.1.10))/(2.1)` [ applying quadratic formula]

`\Rightarrow x=(21\pm21.93)/(2.1)`

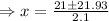
⇒ x= 21.465, -0.465

x= - 0.465 does not possible, since time can not be negative.

∴x=21.465

The hose pipe can fill the pond in 21.465 hours.

The inlet pipe can fill the pond in (21.465-1)=20.465 hours.