Answer:
a) Since we are conducting a left tailed test and the significance level is
 we need to find a critical value on the left tail that accumulate 0.01 of the area on the left and we got
we need to find a critical value on the left tail that accumulate 0.01 of the area on the left and we got

And the rejection zone of the null hypothesis would be
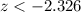
b) For this case since the statistic calculated is -3.33 <--2.326 we have enough evidence to reject the null hypothesis at 1 % of significance for this case.
Explanation:
1) Concepts and formulas to use
We can asume that we need to conduct a hypothesis in order to test the claim that the true proportion is lower than 0.33:
Null hypothesis:
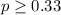
Alternative hypothesis:
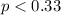
When we conduct a proportion test we need to use the z statisitc, and the is given by:
 (1)
(1)
The One-Sample Proportion Test is used to assess whether a population proportion
 is significantly different from a hypothesized value
is significantly different from a hypothesized value
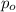 .
.
Calculate the statistic
For this case the statistic calculated is
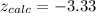
Part a
Since we are conducting a left tailed test and the significance level is
 we need to find a critical value on the left tail that accumulate 0.01 of the area on the left and we got
we need to find a critical value on the left tail that accumulate 0.01 of the area on the left and we got

And the rejection zone of the null hypothesis would be
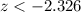
Part b
For this case since the statistic calculated is -3.33 <--2.326 we have enough evidence to reject the null hypothesis at 1 % of significance for this case.