Question

A population of protozoa develops with a constant relative growth rate of 0.6137 per member per day. On day zero the population consists of six members. Find the population size after seven days.

Answer 1

The population size after seven days will be 171.

Explanation:

The population of protozoa can be modeled by the following equation.

`Q(t) = Q(0)(1+r)^(t)`

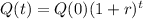
In which Q(t) is the population after t days, Q(0) is the initial population and r is the growth rate.

In this problem, we have that:

`Q(0) = 6, r = 0.6137`

So

`Q(t) = 6(1.6137)^(t)`

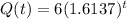
Find the population size after seven days.

This is Q(7).

`Q(7) = 6(1.6137)^(7) = 171`

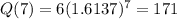
The population size after seven days will be 171.